|
問題3 $xyz$空間の３点 $A(5,0,0),B(4,1,0),C(5,0,\sqrt{2})$ が定める平面を$T$，$T$上にあって点$A$を中心として半径$\sqrt{2}$をもつ円を$U$とする． このとき，以下の問いに答えよ． (1) 点$P$は円$U$の周上にある． $\angle {PAB}=\theta \ \ \ (0 \leqq \theta \text{<}2\pi)$ とするとき,$P$の座標$(u,v,r)$ を$\theta $を用いて表せ． (2) 2$点D(10,0,0),P$を通る直線が$yz$平面と交わる点を$Q(0,Y,Z)$とする．$Y$と$Z$を$\theta $を用いて表せ． (3) (2)の$Y,Z$から$\theta $を消去して，$Q$の軌跡が楕円となることを示せ．またその楕円の概形を$yz$平面上の図示せよ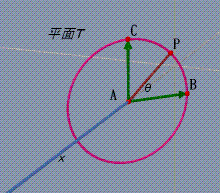 
この問題で，空間における円の取り扱いを学びましょう．なお，この問題で扱う円錐は斜円錐ですが，解説で斜円錐の方程式を求めますが，その方程式は２次式ですから， 斜円錐の平面による切り口の曲線は２次曲線以外現れません． 解答 $(1) \ \ \overrightarrow{AB}=(-1,1,0),\overrightarrow{AC}=(0,0,\sqrt{2})$ より $|\overrightarrow{AB}|=|\overrightarrow{AB}|=\sqrt{2},\overrightarrow{AB}\perp \overrightarrow{AB}$ であるので， $\overrightarrow{OP}=\overrightarrow{OA}+\cos \theta \overrightarrow{AB}+\sin\theta \overrightarrow{AC}$ $=(5-\cos \theta ,\cos \theta ,\sqrt{2}\sin \theta)$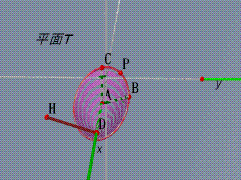 
$(2) \ \ \overrightarrow{DQ}=t\overrightarrow{DP}$より $(-10,Y,Z)=t(-5-\cos \theta ,\cos \theta ,\sqrt{2}\sin \theta)$ したがって， $t=\dfrac{10}{5+\cos \theta},Y=t\cos \theta ,Z=\sqrt{2}t\sin \theta$ $\therefore Y=\dfrac{10\cos \theta}{5+\cos \theta},Z=\dfrac{10\sqrt{2}\sin \theta}{5+\cos \theta}$ $(3) \ \cos \theta ,\sin \theta$を$Y,Z$について解いて $\cos ^2 \theta +\sin ^2 \theta =1$ に代入する． $\cos \theta =\dfrac{5Y}{10-Y},\sin \theta =\dfrac{5Z}{\sqrt{2}(10-Y)}$ より,求める曲線は楕円でその方程式は $Y^2+\dfrac{Z^2}{2}=\dfrac{(10-Y)^2}{25}$ である． 解説 $1. \ \ $円の媒介変数表示について説明を付け加えます． $xy$平面上の原点を中心とする半径1の円は $(\cos \theta ,\sin \theta)$ と表示されます． したがって， $ \begin{pmatrix} \cos \theta \\ \sin \theta \end{pmatrix} =\cos \theta \begin{pmatrix} 1 \\ 0 \end{pmatrix} +\sin \theta \begin{pmatrix} 0 \\ 1 \end{pmatrix} \ \ \cdots \text{@}$ です.@を原点の周りに$\alpha $回転させても変りませんから，原点を中心とする半径1の円は $\begin{pmatrix} \cos \alpha & -\sin \alpha \\ \sin \alpha & \cos \alpha \end{pmatrix} \begin{pmatrix} \cos \theta \\ \sin \theta \end{pmatrix} =\cos \theta \begin{pmatrix} \cos \alpha \\ \sin \alpha \end{pmatrix} +\sin \theta \begin{pmatrix} -\sin \alpha \\ \cos \alpha \end{pmatrix}$ したがって，直交する単位ベクトル$\overrightarrow{u},\overrightarrow{v}$を $\overrightarrow{u}= \begin{pmatrix} \cos \alpha \\ \sin \alpha \end{pmatrix}, \overrightarrow{v}= \begin{pmatrix} -\sin \alpha \\ \cos \alpha \end{pmatrix}$ とおけば，原点を中心とする半径1の円は $\cos \theta \overrightarrow{u}+\sin \theta \overrightarrow{v}$ と表せることが分かりました． 次に@を$x$軸に関する対称移動をすると， $\cos \theta \begin{pmatrix} 1 \\ 0 \end{pmatrix} +\sin \theta \begin{pmatrix} 0 \\ -1 \end{pmatrix} \ \ \ \cdots \text{A}$ Aを回転しても当然原点を中心とする半径1の円ですから，$\overrightarrow{u},\overrightarrow{v}$を逆にとっても かまわないことがわかります．すなわち，原点を中心とする半径１の円は平面上の直交する２つの単位ベクトル $\overrightarrow{u},\overrightarrow{v}$ を用いて， $\cos \theta \overrightarrow{u}+\sin \theta \overrightarrow{v}$ と表せることが分かりました． もちろん $x\overrightarrow{u}+y\overrightarrow{v} \ \ (x^2+y^2=1)$ でもかまいません． なお対応する点は$\theta$が$0$から$2\pi$まで動くとき，$\overrightarrow{u}$の終点から $\overrightarrow{v}$の終点に向かって動き一周します． さらに，$A(\overrightarrow{a})$を中心とする半径$r$の円上の点$P(\overrightarrow{p})$は $\overrightarrow{p}=\overrightarrow{a}+r\cos \theta \overrightarrow{u}+r\sin \theta \overrightarrow{v}$ となります． $2. \ \ $ 空間内の平面上に２つのベクトル$\overrightarrow{u},\overrightarrow{v}$が与えられたとき，直交する単位ベクトルを作るには $\overrightarrow{e_1}=\dfrac{\overrightarrow{u}}{|\overrightarrow{u}|}$ さらに $(t\overrightarrow{u}+s\overrightarrow{v})\cdot \overrightarrow{e_1}=0,|t\overrightarrow{u}+s\overrightarrow{v}|=1$ より$\overrightarrow{e_2}$を求めることになりますが，もしも外積の知識があれば， $\overrightarrow{e_1}=\dfrac{\overrightarrow{u}}{|\overrightarrow{u}|}$ $\overrightarrow{n}=\dfrac{\overrightarrow{u}\times \overrightarrow{u}}{|\overrightarrow{u}\times \overrightarrow{u}|}$ $\overrightarrow{e_2}=\overrightarrow{n}\times \overrightarrow{e_1}$ より得られる$\overrightarrow{e_1},\overrightarrow{e_2}$が平面上の直交する単位ベクトルです．さらに， $\{ \overrightarrow{e_1},\overrightarrow{e_2},\overrightarrow{n}\}$ が与えられた平面の右手系をなす互い直交する単位ベクトルです．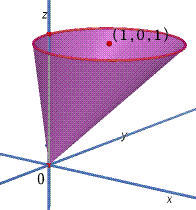 
$3. \ \ $この問題で扱われる円錐は斜円錐です．斜円錐の方程式を求めよう．ここでは，頂点が原点，底面の円が$(x-1)^2+y^2=1,z=1$ である斜円錐の方程式を求める． この斜円錐を平面$z=t$で切ると $(x-t)^2+y^2=t^2,z=t$ $t$を消去して $(x-z)^2+y^2=z^2$ となる．注目することは，$x,y,z$の２次式であることです．一般の斜円錐でも，底面の円が$z=1$上にあるようにとれば， 円の中心と半径が異なるだけですから， ２次式であることが分かります．一方平面の方程式は１次式であるから，斜円錐を平面で切ったときの切り口は２次曲線になります． なお斜円錐は立体図形の入試問題1.問題２および その解説で扱っています． 問題に戻る 目次 全体の目次 |