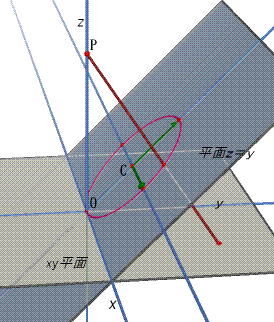
���2�i�_�����̎ˉe�j
��ԓ��̓_�̏W��
$\{ (x,y,z)|0\leqq y,0\leqq z \}$
�Ɋ܂܂�C���_$O$�ɂ�����$x$���ɐڂ��C$xy$���ʂ�$45^{\circ}$�̌X�����Ȃ��C���a�P�̉~��$C$������D
���W��$(0,0,2\sqrt{2})$�̈ʒu�ɂ���_����$P$�ɂ��C$xy$���ʏ�ɓ�����ꂽ�~��$C$�̉e��$S$�Ƃ���D
(1) $S$�̗֊s��\��$xy$���ʏ�̋Ȑ��̕����������߂�D
(2) �~��$C$�Ɖe$S$�̊Ԃɂ͂��܂�C���̓͂��Ȃ������̍�闧�̂̑̐ς����߂�D
 ��
��
����$z=y$���
$(0,\dfrac{1}{\sqrt{2}},\dfrac{1}{\sqrt{2}})$
�𒆐S�Ƃ��锼�a�P�̉~�̎������߂悤�D
����$z=y$��̒�������P�ʃx�N�g��
$\overrightarrow{u}=(1,0,0),\overrightarrow{v}=(0,\dfrac{1}{\sqrt{2}},\dfrac{1}{\sqrt{2}})$
��p����C���̉~����̓_$T(x,y,z)$��
$(x,y,z)=(0,\dfrac{1}{\sqrt{2}},\dfrac{1}{\sqrt{2}})+\cos \theta \overrightarrow{u}+\sin \theta \overrightarrow{v}$
$=(\cos \theta,\dfrac{1+\sin \theta}{\sqrt{2}},\dfrac{1+\sin \theta}{\sqrt{2}})$
���������āC����$PT$��̓_��
$(0,0,2\sqrt{2})+t(\cos \theta,\dfrac{1+\sin \theta}{\sqrt{2}},\dfrac{1+\sin \theta}{\sqrt{2}}-2\sqrt{2})$
$z=0$���$t$�����߂��
$t=\dfrac{4}{3-\sin \theta}$
$S$�̗֊s��\��$xy$���ʏ�̋Ȑ��̔}��ϐ��\���́C
$x=\dfrac{4\cos \theta}{3-\sin \theta},y=\dfrac{4(1+\sin \theta)}{\sqrt{2}(3-\sin \theta)}$
$\sin \theta,\cos \theta$��$x,y$�ɂ��ĉ����C
$\cos ^2 \theta+\sin ^2 \theta=1$
�ɑ�����C
$x^2+y^2-2\sqrt{2}y=0$�E�E�E�@
��D
 (2) ���߂闧�̂̑̐ς́C�Ή~���̂�������$z=y$�����$xy$���ʂɋ��܂ꂽ�����̑̐ςł���D
(2) ���߂闧�̂̑̐ς́C�Ή~���̂�������$z=y$�����$xy$���ʂɋ��܂ꂽ�����̑̐ςł���D
���̎Ή~���̕���$z=y$�̏�̕����̍�����
$\angle POC=\dfrac{\pi}{4}$
���Q�D
�܂��~�@�̔��a��$\sqrt{2}$.
���������ċ��߂�̐ς�
$\dfrac{2\pi \cdot 2\sqrt{2}}{3}-\dfrac{\pi \cdot 2}{3}=\dfrac{(4\sqrt{2}-2)\pi}{3}$
���
��Ԃɂ�����2�~�̊W�͔��]�ϊ���p����ƕ�����Ղ��D
��Ԃɂ����锽�]�ϊ��ɂ��Ă�
��Ԃɂ����锽�]�ϊ�(1)
�̒�`����і���1��ǂ�ł����悢�D
�_$O$�]�̒��S�Ƃ��锽�]�ϊ�$I(O)$�ŋ��ʂ╽�ʂ̑��͋��ʂ╽�ʂł���D
��Ԃɂ�����~�����ʂƕ��ʂ̌���܂���2�̋��ʂ̌���ƌ���C���]�ϊ��ɂ��~�̑��͉~�ł��邱�Ƃ�������D
����ɁC���_$O$�𒆐S�Ƃ���Ή~���̑��ʂ̔��]�ϊ�$I(O)$�ɂ�鑜�͓������ʏ���C
�Ή~���̒�ʂ��~$C$�Ƃ���ƁC$I(O)$�ɂ��~�̑��͂�͂�~�ł���C���̉~�͎Ή~����ł���D�w�i�m���̊T�_�͂��ꂮ�炢�ɂ��Ė��2�������܂��傤�D�T�_�����ł͖��͉����܂���D
 ���2�̕ʉ�
���2�̕ʉ�
�~$C$���܂ޕ���$z=y$��$\alpha$�Ƃ���D
$yz$���ʂ�$\pi$�Ƃ��C����$\pi$��ōl����D
����$\pi$��$\alpha$�ɂ�$xy$���ʂɂ������ł���D
�~$C$�Ɠ_$P(0,0,2\sqrt{2})$���܂ދ��ʂ�$S$�Ƃ���D���̋�$S$�͒��S$S(0,0,\sqrt{2})$���a$\sqrt{2}$�ł���D
�~$C$�͋���$S$�ƕ���$\alpha$�̌���ł���D
$P$�𒆐S�Ƃ��锼�a$2\sqrt{2}$�]���Ƃ��锽�]�ϊ���$I(O,(2\sqrt{2})^2)$�Ƃ���D
���]�ϊ�$I(O,8)$�ɂ��~$C$�̑���$I(O,8)$�ɂ�鋅��$S$�̑���$C$���܂ޕ���$\alpha$�̑��̌���ł���D
����$S$�̑���$xy$���ʂł���D
����$\alpha$�̑������߂�D
$P$���畽��$\alpha$�ɉ��낵�������̑���$A(0,\sqrt{2},\sqrt{2})$.
$I(P,PO^2):A\mapsto B(0,2\sqrt{2},0)$
���������āC����$\alpha$�̑���$A(0,\sqrt{2},\sqrt{2})$�𒆐S�Ƃ��锼�a$2$�̋��D����������
$xy$���ʂƂ̌�������߂�~��
$(\sharp)$��$z=0$������
$x^2+(y-\sqrt{2})^2=2,z=0�@\blacksquare$
�Ή~���Ő�����~�ƂȂ�ꍇ����ʓI�Ȍ`�Ō��Ă݂悤�D
���_��$O$��ʂ��~$C$�̎Ή~����$T$�Ƃ���D
�~$C$(���S��$C$)���܂ޕ��ʂ�$\alpha$,$O$���畽��$\alpha$�ɉ��낵�������̑���$H$,3�_$O,C,H$�̒�߂镽�ʂ�$\pi$�Ƃ���D
���̂Ƃ�$\pi \perp \alpha $�ł���D$(\because OH \subset \pi)$
���̕���$\pi$���~��$T$�̎啽�ʂƂ����D
$CH$�Ɖ~$C$�̌�_��$A,B$�Ƃ���D
����$\pi$��ŁC$\triangle OAB$�̊O�S��$S$�Ƃ���D
����$AB$�̒��_$C$�͉~$C$�̒��S�ŁC$SC\perp \alpha$�ł��邩��$S$�͉~$C$����ѓ_$O$���܂ދ��ʂ̒��S�ł���D�~$C$�Ɠ_$O$���܂ދ��ʂ�$S$�Ƃ���D
�~$C$�͉~��$T$�ƕ���$\alpha$,����$S$�̋��ʂ̌���ł���D
$O$�]�ϊ��̒��S�Ƃ��锽�]�ϊ���$I(O)$�Ƃ���D
$I(O)$�ɂ��C$S$�̑��͕��ʂ�$\alpha$�̑��͋��ʂł��邩��C$C$�̑�$C'$�͉~�ł��邱�Ƃ�������D
���$I(O)$�ɂ��~��$T$�̑���$T$���g�ł���D���������āC�~$C'$�͉~��$T$�ɂ���C$C'$�͉~��$T$��$I(O)$�ɂ�鋅��$S$�̑��ł��镽�ʂƂ̌���ł���D
$I(O)$�ɂ�鋅��$S$�̑������߂�D
���߂鑜�͕��ʂŁC$O$�ɂ����鋅��$S$�Ɛڂ��镽�ʂƕ��s�ł���D���̕��ʂ�$\beta$�Ƃ���.
����$\beta$�͖@���x�N�g����$\overrightarrow{SO}$�ł���@���x�N�g����$\pi$����
$\beta \perp \pi$
����$OA,OB$�ƕ���$\beta$�Ƃ̌�_�����ꂼ��$A',B'$�Ƃ���D
 �_$A',B'$���~$C'$��ł��邱�Ƃ͕����邪�C$A'B'$���~$C'$�̒��a�ł��邱�Ƃ͖��炩�łȂ��D����������D
�_$A',B'$���~$C'$��ł��邱�Ƃ͕����邪�C$A'B'$���~$C'$�̒��a�ł��邱�Ƃ͖��炩�łȂ��D����������D
2����$\alpha,\beta$�̌����$l$�Ƃ���D
$l \perp \pi$
�ł���D$(\because OH\perp l,OS\perp l)$
����������$\alpha$���$l\perp AB$
����$\alpha$��ʼn~$C$��$A$�ɂ�����ڐ���$m$�Ƃ����$m\parallel l$
2����$OA,m$�̒�߂镽�ʂƕ���$\beta$�Ƃ̌����$m'$�Ƃ����$m'\parallel l$�ł��~$C'$
���L�_��$A'$�����ł���D$(\because C,l$�̋��L�_��$A$����$)$
���������āC$m'$�͕���$\beta$��ʼn~$C'$�̓_$A'$�ɂ�����ڐ��D���l��$B'$�ɂ�����~$C'$
�̐ڐ�$n'$��$n'\parallel l$
����������$m'\parallel n'$���$A'B'$���~$C'$�̒��a�ł��邱�Ƃ�������D
�~$C,C'$��$\pi$�ɂ�����ł��钼�a$AB,A'B'$�̊W�ׂ�D
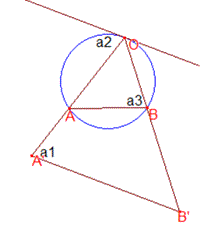 ����$\pi$���
����$\pi$���
$a1=a2=a3$
���4�_$A,A',B',B$�͓���~����ɂ���D����$AB,CD$�̊W��$\angle AOB$�ɂ����ċt���s�ł���Ƃ����D
4�_$A,A',B',B$�̒�߂�~�̒��S��$V$�Ƃ���D���̂Ƃ�
$VC\perp \alpha,VC'\perp \beta$
�ł��邩��C$V$�𒆐S�Ƃ��锼�a$VA$�̋��ʂ�2�~$C,C'$���܂ށD���Ȃ킿�C2�~$C,C'$
�͓��ꋅ�ʏ�ɂ���D
�ȏ�̓��e���܂Ƃ߂�Ǝ��̂悤�ɂȂ�D
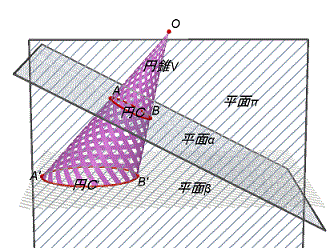 ����
����
���_��$O$��ʂ��~$C$�̎Ή~����$V$�Ƃ��C$V$�̎啽�ʂ�$\pi$�Ƃ���D
$\pi$�Ɖ~$C$�̌�_��$A,B$�Ƃ���D
$V$�ʂŐ���������~�ɂȂ�̂͒��$C$���܂ޕ���$\alpha$�ƕ��s�ȕ��ʂ̑��ɂ������ނ̕��ʂ�����D���̕��ʂ�$\beta$�Ƃ���ƁC$\beta$�͎啽��$\pi$�Ɛ����ŁC$\beta$
��$OA,OB$�Ƃ̌�_�����ꂼ��$A',B'$�Ƃ����4�_$A,A',B',B$��$\pi$��œ���~����ɂ���ꍇ�ł���D�����$V$��$\beta$�̌���ł���~$C'$�̒��a�͐���$A'B'$�ł���D�܂��C2�~$C,C'$�͓��ꋅ�ʏ�ɂ���D
����̍Ō�̕����̋t���Ȃ킿
����
��Ԃɂ����āC2�~$C,C'$�����ꋅ�ʏ�ɂ���C2�~$C,C'$�����ʏ�ɂ���Ή~�������݂���D
�����藧�D
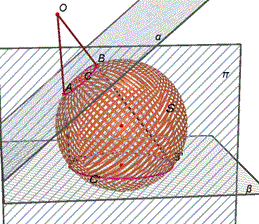 �ؖ�
�ؖ�
2�~$C,C'$������$S$��Ƃ���D$C,C',S$�̒��S�͂��ꂼ�ꓯ������$C,C',S$��p����D
3�_$C,C',S$��ʂ镽�ʂ�$\pi$,�~$C,C'$���܂ޕ��ʂ����ꂼ��$\alpha,\beta$�Ƃ����
$\pi \perp \alpha,\pi\perp \beta\cdots (\sharp)$
$(\because SC \perp \alpha,SC'\perp \beta)$
$\pi$�Ɖ~$C$�Ƃ̌�_��$A,B$,$\pi$�Ɖ~$C'$�Ƃ̌�_��$A',B'$�Ƃ���D
2����$AA',BB'$�̌�_��$O$�Ƃ���D�����C$O$������$S$�̓����Ȃ�C$AB',A'B$�̌�_��$O$
�Ƃ��C$O$�͋���$S$�̊O���Ƃ���D
$\pi$��ŁC4�_$A,A',B',B$�͓���~��ɂ���C����$(\sharp)$�����藧����C
���_$O$,���$C$�̎Ή~���̕���$\beta$�ɂ�����͒��a��$A'B'$�̉~�ŁC����͉~$C'$
�ƈ�v����D���������āC�@$O$�_�C$C$����ʂ̎Ή~���̑��ʏ�ɉ~$C'$�����݂���D$\blacksquare$
(2025�N8������)
����
�ڎ�
�S�̖̂ڎ�
|
 ��
�� (2) ���߂闧�̂̑̐ς́C�Ή~���̂�������$z=y$�����$xy$���ʂɋ��܂ꂽ�����̑̐ςł���D
(2) ���߂闧�̂̑̐ς́C�Ή~���̂�������$z=y$�����$xy$���ʂɋ��܂ꂽ�����̑̐ςł���D ���2�̕ʉ�
���2�̕ʉ� �_$A',B'$���~$C'$��ł��邱�Ƃ͕����邪�C$A'B'$���~$C'$�̒��a�ł��邱�Ƃ͖��炩�łȂ��D����������D
�_$A',B'$���~$C'$��ł��邱�Ƃ͕����邪�C$A'B'$���~$C'$�̒��a�ł��邱�Ƃ͖��炩�łȂ��D����������D ����$\pi$���
����$\pi$��� ����
���� �ؖ�
�ؖ�