|
問題7 $xyz$空間の$zx$平面にある曲線$z=x^2$の，$0\leqq z\leqq h$に対する部分を$C$とする． ただし，$h > 0$である．回転軸$l$を$z$軸にとり，$C$を$l$の周りに１回転させて得られる曲面 からなる容器を$S$とする．$S$に水を満たした後，$S$の回転軸$l$を$z$軸に対して角$\theta$だけ傾ける． 以下$a=\tan \theta$とおく． (1) 水がすべてこぼれず，容器の中に残るための条件は$0\leqq a < ( \ \ \text{ア} \ \ )$である． このとき空気に触れている水面の面積を$T(a)$とすると$T(a)=( \ \ \text{イ} \ )$である． $\displaystyle \lim_{a\to +0}T'(a)=( \ \ \text{ウ} \ \ )$であり，$a$の関数$T(a)$が$0 < a < (\text{ア} )$ の範囲に極大値をもつための条件は$h > ( \ \text{エ} )$である． (2) $a=\sqrt{h}$のとき容器に残った水の体積$V$を求めると$V=(\ \ \text{オ} \ \ )$である．ただし，その計算にあたって，必要ならば 次の定積分を用いてよい． $\displaystyle \int_0^1(1-t^2)^{\frac{3}{2}}dt=\dfrac{3\pi}{16},\int_0^1t^{\frac{3}{2}}(1-t)^{\frac{3}{2}}dt=\dfrac{3\pi}{128}, \int_0^1t^{\frac{3}{2}}(1-t)^{\frac{1}{2}}dt=\dfrac{\pi}{16}$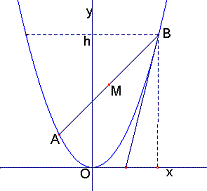 
解答右図を$O$の周りに回転して,$B$における接線が水平になったとき水がなくなり$B$における接線の傾きは$2\sqrt{h}$ であるから $0\leqq a < 2\sqrt{h}$ $S$の回転軸$l$を$z$軸に対して角$\theta$だけ回転して，水がこぼれてから戻す． $S$の軸を含む平面で切った切り口で考える．右図で水面は線分$AB$である． 水面は楕円と仮定して(解説で説明する),長軸と短軸の長さを求める． 直線$AB$の方程式は $z-h=a(x-\sqrt{h})$ であるから，連立方程式 $z=x^2,z-h=a(x-\sqrt{h})$ を解いて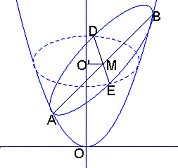 
$A(a-\sqrt{h},(a-\sqrt{h})^2),B(\sqrt{h},h)$したがって， (長軸の長さ)$=AB$ $=\sqrt{1+a^2}(2\sqrt{h}-a)$ 短軸の長さは$M$を通り，回転軸($z$軸)に垂直な平面で切った図形である右図の$DE$ の長さである． $O'D^2=(M$の$y$座標$)=\dfrac{(a-\sqrt{h})^2+\sqrt{h}^2}{2}$ したがって， $MD^2=\dfrac{(a-\sqrt{h})^2+(\sqrt{h})^2}{2}-\dfrac{a^2}{4}=\dfrac{(2\sqrt{h}-a)^2}{4}$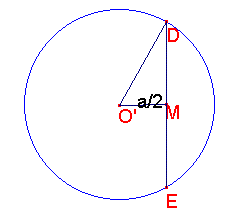 
空気に触れている水面の面積$T(a)$は$T(a)=\pi \cdot \dfrac{AB}{2}\cdot MD=\dfrac{\pi (2\sqrt{h}-a)^2\sqrt{1+a^2}}{4}$ $T'(a)=\dfrac{\pi (2\sqrt{h}-a)}{4\sqrt{1+a^2}}(-3a^2+2\sqrt{h}a-2)$ より $a\to +0$のとき$T'(a)\to -\pi \sqrt{h}$ $f(a)=-3a^2+2\sqrt{h}a-2=-3(a-\dfrac{\sqrt{h}}{3})^2+\dfrac{h}{3}-2$ であり， $f(0)=-2 < 0,f(2\sqrt{h})=-8h-2 < 0$ さらに $0 < \dfrac{\sqrt{h}}{3} < 2\sqrt{h}$ より $f(\dfrac{\sqrt{h}}{3})=\dfrac{h}{3}-2 > 0 \ \ \ \therefore h > 6$ 
(2)水面は$B$を通る傾き$\sqrt{h}$であるから原点を通る．放物線$z=x^2$を$z$軸の周りに回転した曲面の式は $z=t$でこの曲面を切ると $x^2+y^2=t,z=t$ であるから，$t$を消去して $x^2+y^2=z$ 一方水面の式は $z=\sqrt{h}x$ である．体積を求める方法は，各座標軸に垂直な平面で切る場合が考えられ３通りある． すべての方法で求めてみよう． (方法1) $x$軸に垂直な平面で切る方法． 水のある部分は$x^2+y^2\leqq z \leqq \sqrt{h}x$ であ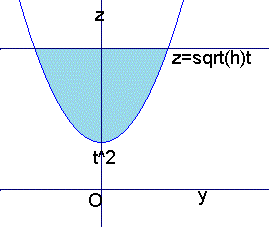る． 
求める領域を$x=t \ (0\leqq t\leqq \sqrt{h})$で切ったとき，切り口の図形を
$yz$平面で考える．$t^2+y^2\leqq z \leqq \sqrt{h}t$ $z=y^2+t^2$と$z=\sqrt{h}t$の交点の$y$座標は $y=\pm \sqrt{\sqrt{h}t-t^2}$ 右側の水色の面積は $\dfrac{(2\sqrt{\sqrt{h}t-t^2})^3}{6}=\dfrac{4}{3}\cdot t^{\frac{3}{2}}(\sqrt{h}-t)^{\frac{3}{2}}$ 求める体積は $\displaystyle \int_0^{\sqrt{h}}\dfrac{4}{3}\cdot t^{\frac{3}{2}}(\sqrt{h}-t)^{\frac{3}{2}}dt$ ここで,問題文にある，積分の値を使うため変形する． $\sqrt{h}-t=\sqrt{h}(1-\dfrac{t}{\sqrt{h}})$ より $u=\dfrac{t}{\sqrt{h}}$と置換する $\displaystyle =\dfrac{4}{3}\int_0^1(\sqrt{h}u)^{\frac{3}{2}}(\sqrt{h}-\sqrt{h}u)^{\frac{3}{2}}\sqrt{h}du$ $=\dfrac{4}{3}h^2\cdot \dfrac{3\pi}{128}=\dfrac{\pi h^2}{32}$ (方法2) $y$軸に垂直な平面で切る方法 水のある領域を$y=t$で切ったとき，切り口の図形を $zx$平面で考える． $x^2+t^2\leqq z \leqq \sqrt{h}x$ $z=x^2+t^2,z=\sqrt{h}x$が接するのは $x^2+t^2=\sqrt{h}x$の判別式を$D$とすると $D=h-4t^2$ より $t^2=\dfrac{h}{4}$ $-\dfrac{\sqrt{h}}{2}\leqq t\leqq \dfrac{\sqrt{h}}{2}$ $z=x^2+t^2$と$z=\sqrt{h}x$で囲まれた面積を$S(t)$とおく． $x^2+t^2=\sqrt{h}x$の解を$\alpha ,\beta$とおくと $\alpha +\beta =\sqrt{h},\alpha \beta =t^2$ より $(\alpha -\beta )^2=h-4t^2$ したがって $S(t)=\dfrac{(h-4t^2)^{\frac{3}{2}}}{6}$ $\displaystyle V=2\int_0^{\frac{\sqrt{h}}{2}}S(t)dt=\dfrac{1}{3}\int_0^{\frac{\sqrt{h}}{2}}(h-4t^2)^{\frac{3}{2}}dt$ ここで $(h-4t^2)^{\frac{3}{2}}=h^{\frac{3}{2}}(1-\dfrac{4t^2}{h})^{\frac{3}{2}}$ と変形し， $u=\dfrac{2t}{\sqrt{h}}$ と置換すると $\displaystyle V=\dfrac{1}{3}h^{\frac{3}{2}}\int_0^1(1-u^2)^{\frac{3}{2}}\dfrac{\sqrt{h}}{2}du=\dfrac{\pi h^2}{32}$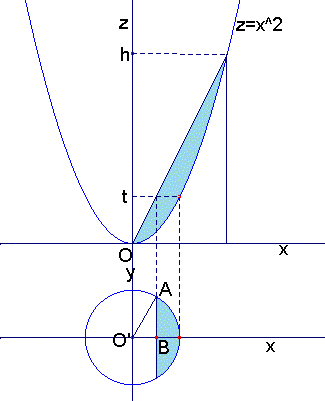 
(方法3) $z$軸に垂直な平面で切る方法水のある領域を$z=t$で切ったとき，切り口の図形を $xy$平面で考える． 水面を表す直線は$y=\sqrt{h}x$であるから右図で $OA=\sqrt{t},OB=\dfrac{t}{\sqrt{h}}$ ここで$\angle AOB=\theta$とおくと $\cos \theta =\dfrac{\sqrt{t}}{\sqrt{h}} \ \ \therefore t=h\cos ^2\theta \cdots (\sharp)$ 水がある弓形の部分の面積$S(t)$を求めると $S(t)=\dfrac{1}{2}\cdot (\sqrt{t})^2\cdot 2\theta -\dfrac{1}{2}\cdot (\sqrt{t})^2\sin 2\theta$ $=t\theta -t\sin \theta \cos \theta$ $\int_0^hS(t)dt$を計算するわけですが，$\theta $を$t$で表せれば問題ないのですが，そのためには三角関数の逆関数が必要です． 高校では学ばないので，$t$を$\theta$を用いて表し，すなわち置換積分で計算します(問題2の解説参照)． $\dfrac{dt}{d\theta}=-2h\cos \theta \sin \theta$ $t:0\to h$のとき$\theta :\frac{\pi}{2}\to 0$ より $\displaystyle \int_0^ht\theta dt =\int_{\frac{\pi}{2}}^0h\cos ^2\theta \cdot \theta \cdot -2h\sin \theta \cos \theta d\theta$ $\displaystyle =2h^2\int_0^{\frac{\pi}{2}}\theta \cos ^3\theta \sin \theta d\theta$ $\displaystyle =2h^2\left(\left[\theta \cdot -\dfrac{\cos ^4\theta}{4}\right]_0^{\frac{\pi}{2}} +\dfrac{1}{4}\int_0^{\frac{\pi}{2}}\cos ^4\theta d\theta \right)$ $=2h^2\cdot \dfrac{1}{4}\cdot \dfrac{3}{4}\cdot \dfrac{1}{2}\cdot \dfrac{\pi}{2}=\dfrac{3\pi h^2}{32}$ $\displaystyle \int_0^ht\sin \theta \cos \theta dt=\int_{\frac{\pi}{2}}^0h\cos ^2\theta \sin \theta \cos \theta \cdot -2h\sin \theta \cos \theta d\theta$ $\displaystyle =2h^2\int_0^{\frac{\pi}{2}}\cos ^4\theta \sin ^2\theta d\theta$ $\displaystyle =2h^2\int_0^{\frac{\pi}{2}}(\cos ^4\theta -\cos ^6\theta)d\theta$ $=2h^2(\dfrac{3}{4}\cdot \dfrac{1}{2}\cdot \dfrac{\pi}{2}-\dfrac{5}{6}\cdot \dfrac{3}{4}\cdot \dfrac{1}{2}\cdot \dfrac{\pi}{2})$ $=\dfrac{\pi h^2}{16}$ したがって， $\displaystyle \int_0^hS(t)dt=\dfrac{3\pi h^2}{32}-\dfrac{\pi h^2}{16}=\dfrac{\pi h^2}{32}$ 続く(解説) 問題に戻る 目次 全体の目次 |