|
問題6(四面体の正射影) 四面体$ABCD$は $AC=\dfrac{\sqrt{3}}{2},AD=1,BC=\dfrac{1}{2}$ であり，辺$AD$は面$ABC$に垂直であり，辺$BC$は 面$ACD$に垂直である． 四面体$ABCD$から十分離れたところに直線$AB$と平行な平面$\alpha$を一つとる．$\alpha$に垂直な平行光線を四面体$ABCD$ にあてて，$\alpha$上に影をつくる．その影の面積を$S$とする． 直線$AB$を軸として四面体$ABCD$を１回転させるとき，$S$の最大値，最小値を求めよ．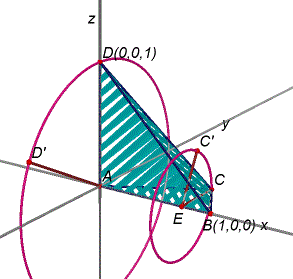 
解答まず辺$AB$の長さを求める． $BC\perp \triangle ACD$より$\angle ACB=\dfrac{\pi}{2}$ したがって $AB=1$ $AD\perp \triangle ABC$より頂点$A$を原点，頂点$B$を$x$軸上，$\triangle ABC$を$xy$ 平面上，$D$を$z$軸上にとって良い． $A(0,0,0),B(1,0,0),D(0,0,1)$ であり，点$C$の座標は$\angle CAB=\dfrac{\pi}{6}$より $C(\dfrac{3}{4},\dfrac{\sqrt{3}}{4},0)$ である． 平面$\alpha$は$xy$平面としてよい． $x$軸の周りに$\theta$回転する変換を $(x,y,z)\mapsto (x',y',z')$ とすると $x'=x,y'+iz'=(y+iz)(\cos \theta +i\sin \theta)$ より $(x,y,z)\mapsto (x,y\cos \theta-z\sin \theta ,y\sin \theta +z\cos \theta)$ である．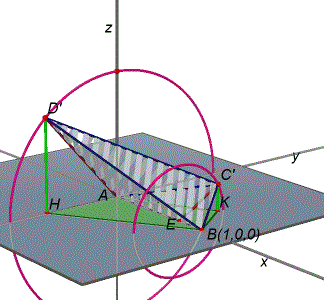 
この変換で$A,B$は動かさず$C(\dfrac{3}{4},\dfrac{\sqrt{3}}{4},0)\mapsto C'(\dfrac{3}{4},\dfrac{\sqrt{3}\cos \theta}{4},\dfrac{\sqrt{3}\sin \theta}{4})$ $D(0,0,1)\mapsto D'(0,-\sin \theta,\cos \theta)$, である． $\theta$と$\theta +\pi$では， $\sin\theta,\cos \theta$の符号が変わるだけで正射影の面積は変わらないから，$0\leqq \theta \leqq 180^{\circ}$ で考えればよい． $C',D'$の$xy$平面への正射影をそれぞれ$K,H$とすると，四面体の正射影は４点$A,H,B,K$ の作る図形である． ４点$A,B,H,K$の$xy$平面での座標はそれぞれ $A(0,0),B(1,0)$ $K(\dfrac{3}{4},\dfrac{\sqrt{3}\cos \theta}{4})$ $H(0,-\sin \theta)$ である． まず，$AB$に関して２頂点$K,H$が同じ側にある場合と反対側にある場合があるのでその場合分けが 必要である． $\bullet \ 0\leqq \theta \leqq \dfrac{\pi}{2}$のとき $S=\dfrac{1}{2}(\dfrac{\sqrt{3}}{4}\cos \theta +\sin \theta )$ 最大値は $\dfrac{1}{2}\sqrt{(\dfrac{\sqrt{3}}{4})^2+1^2}=\dfrac{1}{2}\cdot \dfrac{\sqrt{19}}{4}$ 最小値は $S(0)=\dfrac{\sqrt{3}}{8},S(\dfrac{\pi}{2})=\dfrac{1}{2}$ より$\dfrac{\sqrt{3}}{8}$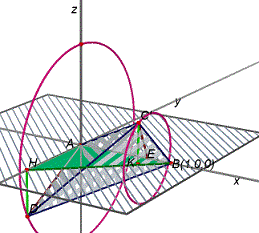 
$\bullet \ \dfrac{\pi}{2}\leqq \theta \leqq \pi$のとき$K$が$\triangle AHB$の中の場合と外の場合に 場合分けが必要（右の２つの図）． $K$が$BH$上のときの$\theta$の値は $AH:EK=1:\dfrac{1}{4}$ より $\theta =\dfrac{2\pi}{3}$ $(a) \ \dfrac{\pi}{2}\leqq \theta \leqq \dfrac{2\pi}{3} \ $のとき このとき，射影は$\triangle AHB$ したがって $S=\dfrac{\sin \theta}{2}$ より $\dfrac{\sqrt{3}}{4}\leqq S\leqq \dfrac{1}{2}$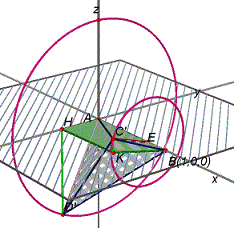 
$(b) \ \dfrac{2\pi}{3}\leqq \theta \leqq \pi \ $のとき射影は四角形$AHKB$ $S=\dfrac{1}{2}(\dfrac{3}{4}\sin \theta -\dfrac{\sqrt{3}}{4}\cos \theta)$ $=\dfrac{\sqrt{3}}{4}\sin (\theta -\dfrac{\pi}{6})$ より $\dfrac{\sqrt{3}}{8}\leqq S\leqq \dfrac{\sqrt{3}}{4}$ 以上の議論より， 最大値は$\dfrac{\sqrt{19}}{8}$でその時の$\theta$は $\sqrt{3}\cos \theta +4\sin \theta \ \ (0\leqq \theta \leqq \dfrac{\pi}{2})$ の最大値を与える$\theta$．この値を求める必要はないと思うが $\sqrt{3}\cos \theta +4\sin \theta =(\sqrt{3},4)\cdot (\cos \theta,\sin \theta)$ より $(\sqrt{3},4)\parallel (\cos \theta,\sin \theta)$ のとき最大値をもつから， $\tan \theta =\dfrac{4}{\sqrt{3}}$ 最小値は$\dfrac{\sqrt{3}}{8}$でその時の$\theta$の値は$0,\pi$である．
$S$の増減を表にすると次のようになる． |