|
例題 極方程式 $r=|\sin 2\theta|$ の表す曲線を直交座標を用いた平面上で考える． (1) この曲線上の点の直交座標を$(x,y)$とすると，$x=( \ \ ),y=( \ \ )$のとき$x$は最大値をとる． (2) この曲線上の点$(x,y)\neq (0,0)$における接線の傾きが１であるとする．この点の偏角を$\theta$とすると， $\tan \theta =( \ \ )$である． (3) この曲線上で点を動かすとき，原点$O$からの距離$r$が$P(x_0,y_0) \ (x_0\text{＞},y_0\text{＞}0)$で 最大値をとるとする．点$P$とは異なるこの曲線上の点$Q(x_1,y_1)$において$x_1=x_0$であるとき，点$Q$の偏角を $\theta$とすると，$\tan \theta =( \ \ )$である． (4) この曲線の概形を直交座標を用いた平面上に図示しなさい（$x$および$y$が最大値，最小値をとる点の座標を記入しなさい）． さらに同一平面上に関数$y=-x+\frac{3+\sqrt{3}}{4}$のグラフを書きなさい（この関数のグラフと上の曲線の交点の座標を 記入しなさい）． (5) この曲線で囲まれた図形と３つの不等式 $x \geqq 0,y \geqq 0,y\leqq -x+\dfrac{3+\sqrt{3}}{4}$ を同時に満たす領域の共通部分の面積を求めなさい． 解答の前に，極座標の復習をします．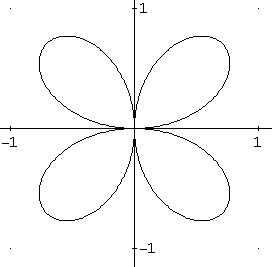 
極方程式$r=f(\theta)$ が与えれれた時， $x,y$の関係式を求めることも， $x=f(\theta)\cos \theta,y=f(\theta)\sin \theta$ と媒介変数表示することも必要ですが，まず最初にやることは，$\theta$の増加により$r$がどのように変化するかを 調べ，概形を書くことです． 実際 $r=\sin 2\theta$ のグラフを書いてみましょう． なお，曲線 $r=\sin n\theta$ を正葉曲線といいます． $\theta$が$0$から$\frac{\pi}{4}$まで増加するとき，$r$も増加し，$\frac{\pi}{4}$から$\frac{\pi}{2}$まで， 増加するとき$r$は減少します． さらに，$\theta$が$\frac{\pi}{2}$から$\pi$まで増加するときは$r$が負の数ですので，第４象限にグラフが現れます． 原点に関する対称移動を$-\pi$の回転と考えれば第１象限 のグラフを原点の周りに$-\frac{\pi}{2}$回転したグラフになります． $\pi \leqq \theta \leqq 2\pi$も同様に調べることにより，右の図になります． グラフは滑らかですから，グラフ上を鉛筆でなぞれば，第１象限，第４象限，第３象限，第２象限と移動することが 分かるでしょう． 次に $r=|\sin 2\theta|$ を考えましょう． $r \geqq 0$であるから，$\theta$が$0$から$2\pi$まで増加するとき，グラフは 第１象限，第２象限，第３象限，第４象限と順に現れ，第１象限のグラフを原点の周りにそれぞれ $\frac{\pi}{2},\pi,\frac{3\pi}{2}$だけ回転した図形になっています． グラフから分かるように$\theta =\frac{\pi}{2},\pi,\frac{3\pi}{2}$では微分可能ではありません．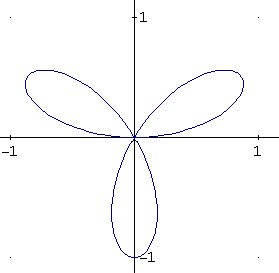 
さて，正葉形$r=\sin n\theta$ について$n$が偶数と奇数では様子が異なります． ここでは$n$が奇数の場合として $r=\sin 3\theta$ を調べます．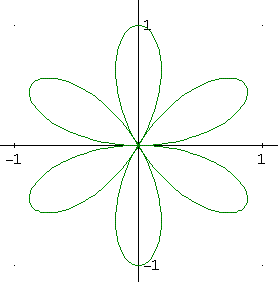 
この場合は３つの葉っぱしかありません．$\pi \leqq \theta \leqq 2\pi$のグラフは，$0 \leqq \theta \leqq \pi$のグラフを繰り返します． $f(\theta +\pi)=-f(\theta)$ が成り立つからです． $r=|\sin 3\theta |$ はどうかというと，これは重ならず６つの葉っぱが現れも右図のようになります． このように，極方程式で与えれたとき，すぐに直交座標に直すのではなく，$\theta$の変化で$r$がどうなるかを調べることが 大切です． それでは解答に入りましょう． 解答 曲線 $r(\theta)=|\sin 2\theta|$ のグラフが$x$軸$y$軸，直線$y=x$に関して対称であることを確認する．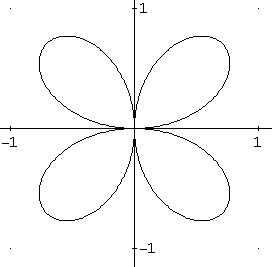
$r(-\theta)=r(\theta)$より$x$軸に関して対称． $r(\dfrac{\pi}{2}+\theta)=r(\dfrac{\pi}{2}-\theta)$ より$y$軸に関して対称. $r(\dfrac{\pi}{4}+\theta)=r(\dfrac{\pi}{4}-\theta)$ より直線$y=x$に関して対称． したがって，$0 \leqq \theta \leqq \dfrac{\pi}{2}$で調べればより． (1) $x=r\cos \theta =\sin 2\theta \cos \theta$ より， $x'(\theta)=-6\cos \theta (\sin ^2 \theta -\dfrac{1}{3})$ したがって，$\alpha$を$\sin \alpha =\dfrac{1}{\sqrt{3}} \ \ (0\leqq \alpha \leqq \dfrac{\pi}{2})$で定めれば， $\theta =\alpha$で$x$は最大値をとる． そのとき $x=\sin 2\alpha \cos \alpha=\dfrac{4}{3\sqrt{3}}$ $y=\sin 2\alpha \sin \alpha=\dfrac{2\sqrt{2}}{3\sqrt{3}}$ あとは対称性を考えることより， $(x,y)=(\dfrac{4}{3\sqrt{3}},\pm \dfrac{2\sqrt{2}}{3\sqrt{3}})$. (2) $0\leqq \theta \leqq \dfrac{\pi}{2}$のとき， $x=\sin 2\theta \cos \theta ,y=\sin 2\theta \sin \theta $ $\dfrac{\pi}{2} \leqq \theta \leqq \pi$のとき， $x=-\sin 2\theta \cos \theta ,y=-\sin 2\theta \sin \theta $ であるから，$0 \leqq \theta \leqq \pi$で， $\dfrac{dy}{dx}=\dfrac{y'(\theta)}{x'(\theta)}$ が成り立つ． $\dfrac{y'(\theta)}{x'(\theta)}=1$ より $x'(\theta )=y'(\theta)$ $\therefore 2\cos 2\theta \cos \theta -\sin 2\theta \sin \theta =2\cos 2\theta \sin \theta +\sin 2\theta \cos \theta $ 両辺を$\cos 2\theta \cos \theta$で割ると， $\tan 2\theta +2\tan 2\theta+2\tan \theta -2=0$ $\tan 2\theta$を$\tan \theta$で表して整理すると， $\tan ^3 \theta -2\tan ^2 \theta -2\tan \theta +1=0$ $\tan \theta =-1$は解である(グラフより$\theta =\dfrac{3\pi}{2}$が解であることは分かる). $\therefore \tan \theta =-1,\dfrac{3\pm \sqrt{5}}{2}$ (3) $0\leqq \theta \leqq \dfrac{\pi}{2}$で $r=\sin 2\theta$ を最大にする$\theta $は$\theta =\dfrac{\pi}{4}$. $x=\sin 2\theta \cos \theta =x(\dfrac{\pi}{4})$ を解く． $\sin 2\theta \cos \theta =\dfrac{1}{\sqrt{2}}$ $\therefore 4\sin ^3 \theta -4\sin \theta +\sqrt{2}=0$ この方程式は，作りかたより$\theta =\dfrac{\pi}{4}$を解にもつことに注目し， $(2\sin \theta -\sqrt{2})(2\sin ^2 \theta +\sqrt{2}\sin \theta -1)=0$ 第１象限で考えているから，$\sin \theta \geqq 0$より $\sin \theta =\dfrac{\sqrt{2}}{2},\dfrac{\sqrt{10}-\sqrt{2}}{4}$ $\sin \theta =\dfrac{\sqrt{2}}{2}$のとき$\tan \theta =1$ 
$\sin \theta =\dfrac{\sqrt{10}-\sqrt{2}}{4}$のとき$\tan \theta =\sqrt{\sqrt{5}-2}$.グラフの対象性より，求める$\tan \theta $の値は， $\tan \theta =1,\pm \sqrt{\sqrt{5}-2}$ (4) (1)でもとめた座標および対称性より，$x$座標が最大，最小である点は $(\pm \dfrac{4}{3\sqrt{3}},\pm \dfrac{2\sqrt{2}}{3\sqrt{3}})$ であり，$y$座標が最大，最小である点は $(\pm \dfrac{2\sqrt{2}}{3\sqrt{3}},\pm \dfrac{4}{3\sqrt{3}})$ である． 次に，曲線 $r=|\sin 2\theta|$ と直線 $x+y=\dfrac{3+\sqrt{3}}{4}$ の交点を求める． 設問(1)から(3)までの問いの答えの中に直線 $x+y=\dfrac{3+\sqrt{3}}{4}$ 上の点があるのかと考えるが，そうではない．交点を新しく求める必要がある． 直線 $x+y=\dfrac{3+\sqrt{3}}{4}$ は極座標に直すと， $r\cos (\theta -\dfrac{\pi}{4})=\dfrac{3+\sqrt{3}}{4\sqrt{2}}$ であるから，$r$を消去すると $\sin 2\theta \cos (\theta -\dfrac{\pi}{4})=\dfrac{3+\sqrt{3}}{4\sqrt{2}}$ である．これを$\sin \theta$や$\cos \theta$の３次式にしても手の施しようがない． $\cos \dfrac{\pi}{12}=\dfrac{\sqrt{3}+1}{2\sqrt{2}}$ であることに注目し， $\theta =\dfrac{\pi}{6}$ が解の１つであることが分かる．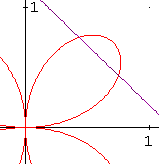 
グラフの対称性より，交点は$\theta =\dfrac{\pi}{6},\dfrac{\pi}{3}$のときで，交点の座標は$A(\dfrac{3}{4},\dfrac{\sqrt{3}}{4}),B(\dfrac{\sqrt{3}}{4},\dfrac{3}{4})$ である． 直線$OA$と曲線で囲まれた図形の面積は $\displaystyle \dfrac{1}{2}\int_0^{\frac{\pi}{6}}r^2d\theta=\dfrac{\pi}{24}-\dfrac{\sqrt{3}}{32}$ $\triangle OAB=\dfrac{3}{16}$ したがって，求める面積は $2(\dfrac{\pi}{24}-\dfrac{\sqrt{3}}{32})+\dfrac{3}{16}=\dfrac{\pi}{12}+\dfrac{3-\sqrt{3}}{16}$ 戻る 目次 全体の目次 |