正$n$角形の作図問題
前章では作図の基本事項を学びました.ここでは具体的問題で,作図が可能かどうかを知らべます.
 まず正$n$角形の作図から始めます.
まず正$n$角形の作図から始めます.
正$n$角形が作図できるための必要十分条件は$\cos \frac{2\pi}{n}$が作図できる.
が成り立つ.
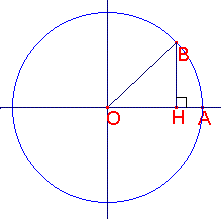
実際,$\cos \frac{2\pi}{n}$が作図できるとき,単位円の一つの半径$OA$
上に
$OH=\cos \dfrac{2\pi}{n}$
を満たす点を$H$をとり,$H$を通り$OA$に垂直な直線と円との交点を$B$
とする.あとは$AB$の幅で円を分ければ正$n$角形ができる.
逆に,正$n$角形が作図できるとき,一つの頂点$B$から隣の頂点を中心を結ぶ半径に垂線
を下しその足を$H$とすれば,
$OH=\cos \dfrac{2\pi}{n}$
であるので$\cos \frac{2\pi}{n}$は作図できる.
まず正17角形が作図できることを示そう.
すでに計算してあり
$\cos \theta =\cos \dfrac{2\pi}{17}=\dfrac{2c+\sqrt{-4c^2+4d+8}}{4}$
$=\dfrac{c+\sqrt{-c^2+d+2}}{2}$
ここで
$t=a=\dfrac{-1+\sqrt{17}}{4},$
$c=\dfrac{t+\sqrt{t^2+1}}{2}
=\dfrac{-1+\sqrt{17}+\sqrt{34-2\sqrt{17}}}{8}$
$d=\dfrac{t-\sqrt{t^2+1}}{2}
=\dfrac{-1+\sqrt{17}-\sqrt{34-2\sqrt{17}}}{8}$
である.
作図可能であることを示すだけなら,これ以上計算する必要はないが,$c,d$
を代入すると
$\cos \dfrac{2\pi}{17}=\dfrac{-1+\sqrt{17}+\sqrt{34-2\sqrt{17}}}{16}$
$+\dfrac{\sqrt{17+3\sqrt{17}-\sqrt{34-2\sqrt{17}}-2\sqrt{34+2\sqrt{17}}}}{8}$
である.
正17角形の作図は前章で述べた作図の可能性の議論は必要ない.
$\sqrt{17},t,\sqrt{t^2+1},c,d,\sqrt{-4c^2+4d+8},\cos \dfrac{2\pi}{17}$
と作図していけばよい.
正$n$角形が作図できるための必要十分条件は$\cos \frac{2\pi}{n}$が作図できる.
にしたがって,正多角形が作図できるかどうかを調べていこう.
$n=3,4,6$のときは作図できる.
実際
$\cos \dfrac{2\pi}{3}=-\dfrac{2}{1},\cos \dfrac{2\pi}{4}=0,\cos \dfrac{2\pi}{6}=\dfrac{1}{2}$
正$n$角形が作図できるとき,
$\cos \dfrac{2\pi}{n}$が作図できる.このとき
$\cos ^2\dfrac{2\pi}{2n}=\dfrac{1+\cos \dfrac{2\pi}{n}}{2}$
であるから,正$2n$角形も作図できる.これは,角の2等分線より容易に分かる.
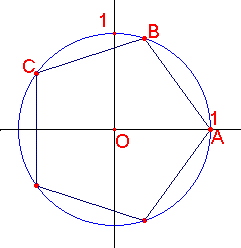
次に$n=5$について考える.
$\theta =\dfrac{2\pi}{5}$と置き,$\cos \theta$をもとめる.
一般には,$5\theta =2\pi$より
$\cos 2\theta =\cos 3\theta$
となるので,$\cos \theta$の3次方程式を作り解く.式を見れば
分かるように$\theta =0$は条件を満たすから$\cos 0=1$は解である.したがって
2次方程式になり作図できることがわかる.
 ここでは,$n=17$でとった方法にしたがって求める.
ここでは,$n=17$でとった方法にしたがって求める.
$\cos \theta +\cos 2\theta =-\dfrac{1}{2}$
$\cos \theta \cos 2\theta =\dfrac{1}{2}(\cos \theta +\cos 3\theta)$
$=\dfrac{1}{2}(\cos \theta +\cos 2\theta)=-\dfrac{1}{4}$
したがって,$\cos \theta$は2次方程式
$x^2+\dfrac{1}{2}x-\dfrac{1}{4}=0$
の解で正の方である.したがって
$x=\cos \dfrac{2\pi}{5}=\dfrac{-1+\sqrt{5}}{4}$
この値は作図できるので正5角形は作図できることがわかる.
正5角形の作図法はいくつかあるが,単位円を描いてこの値を使う
ことが分かり易い作図方法である.
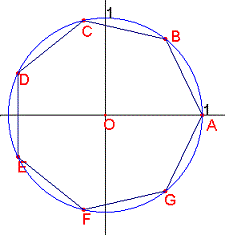 次に$n=7$について考える.
次に$n=7$について考える.
$\theta =\dfrac{2\pi}{7}$と置き,$\cos \theta$をもとめる.
ここでも$n=17$でとった方法にしたがって求める.
$\cos \theta +\cos 2\theta +\cos 3\theta =-\dfrac{1}{2}$
$4(\cos \theta \cos 2\theta)\cos 3\theta $
$=2(\cos \theta +\cos 3\theta)\cos 3\theta$
$=\cos 2\theta +\cos 4\theta +1+\cos 6\theta $
$=\cos \theta +\cos 2\theta +\cos 3\theta +1$
$=\dfrac{1}{2}$
$2(\cos \theta \cos 2\theta +\cos \theta \cos 3\theta +\cos 2\theta \cos 3\theta)$
$=\cos \theta +\cos 3\theta +\cos 2\theta +\cos 4\theta +\cos \theta +\cos 5\theta $
$=2(\cos \theta +\cos 2\theta +\cos 3\theta )$
$=-1$
したがって,$\cos \theta,\cos 2\theta ,\cos 3\theta$を解とする3次方程式は
$x^3+\dfrac{1}{2}x^2-\dfrac{1}{2}x-\dfrac{1}{8}=0$
すなわち
$8x^3+4x^2-4x-1=0\cdots (\sharp)$
の解である.
整数係数の3次方程式の解は,有理数でなければ作図できないことが証明されている.
$(\sharp)$が有理数解をもつかどうかを調べよう.
解になり得る有理数は
$\pm \dfrac{1}{8},\pm\dfrac{1}{4},\pm \dfrac{1}{2},\pm 1$
だけでそれを調べてもよいが,
$y=2x$とおくと$(\sharp)$は
$y^3+y^2-2y-1=0\cdots (\flat)$
となり,この方程式で解になり得る有理数は$\pm 1$だけで,$\pm 1$を代入すると,左辺は奇数であるので$0$には
ならない.したがって,有理数解をもたないことが分かった.
これで正7角形が作図できないことが証明された.
$\cos \frac{2\pi}{7}$が満たす3次方程式は次のようにして求めるのが一般的です.
(別方法1)
$\theta =\dfrac{2\pi}{7}$とおく.
$7\theta =2\pi$より
$\cos 4\theta =\cos 3\theta$
さらに
$\cos 4\theta =8\cos ^4\theta -8\cos ^2\theta +1$
$\cos 3\theta =4\cos ^3\theta -3\cos \theta$
より
$8\cos ^4\theta -8\cos ^2\theta +1=4\cos ^3\theta -3\cos \theta$
$x=\cos \theta$とおくと
$8x^4-4x^3-8x^2+3x+1=0$
$\theta =0$は条件を満たすから$x=1$は解である.
$(x-1)(8x^3+4x^2-4x-1)=0$
$\therefore 8x^3+4x^2-4x-1=0$
(別方法2)
$z^7=1$
を解く.
$(z-1)(z^6+z^5+z^4+z^3+z^2+z+1)=0$
$z^6+z^5+z^4+z^3+z^2+z+1$
は相反方程式であるから$z^3$で割り
$(z^3+\dfrac{1}{z^3})+(z^2+\dfrac{1}{z^2})+(z+\dfrac{1}{z})=0$
$(z+\dfrac{1}{z})^3+(z+\dfrac{1}{z})^2-2(z+\dfrac{1}{z})-1=0$
$x=z+\dfrac{1}{z}$とおくと
$x^3+x^2-2x-1=0$
一方,$z$は$z^7=1$より$|z|=1$
$z=\cos \theta +i\sin \theta$
とおくと
$z+\dfrac{1}{z}=z+\bar{z}=2\cos \theta$
したがって,$\cos \theta$は
$(2\cos \theta)^3+(2\cos \theta)^2-2(2\cos \theta)-1=0$
を満たす.
続く(ギリシャの三大作図問題)
目次
|  まず正$n$角形の作図から始めます.
まず正$n$角形の作図から始めます. ここでは,$n=17$でとった方法にしたがって求める.
ここでは,$n=17$でとった方法にしたがって求める. 次に$n=7$について考える.
次に$n=7$について考える.