$\S11.$シェルク極小曲面 正則関数$\phi (w)$を定め，$F(w),G(w),H(w)$を $\displaystyle F(w)=\dfrac{1}{2}\int (1-w^2)\phi (w)dw,$ $\displaystyle G(w)=\dfrac{i}{2}\int (1+w^2)\phi (w)dw,$ $\displaystyle H(w)=\int w\phi (w)dw$ によって定義する．このとき，$F,G,H$の実部，虚部により定義された曲面 $\boldsymbol{p}(u,v)=(\mathcal{Re}(F(w)),\mathcal{Re}(G(w)),\mathcal{Re}(H(w)) =(x(u,v),y(u,v),z(u,v))$ $\boldsymbol{q}(u,v)=(\mathcal{Im}(F(w)),\mathcal{Im}(G(w)),\mathcal{Im}(H(w)) =(\bar{x}(u,v),\bar{y}(u,v),\bar{z}(u,v))$ はともに極小曲面である． ここでは$\phi (w)=\dfrac{4}{1-w^4}$の場合を考える． $\displaystyle F(w)=\dfrac{1}{2}\int \dfrac{4(1-w^2)}{1-w^4}dw =2\int \dfrac{dw}{1+w^2}=2\arctan w$ $\displaystyle G(w)=\dfrac{i}{2}\int \dfrac{4(1+w^2)}{1-w^4}dw =2i\int \dfrac{dw}{1-w^2}=2i\dfrac{\arctan iw}{i}=2\arctan (iw)$ $\displaystyle H(w)=\int \dfrac{4w}{1-w^4}dw$ $t=w^2$とおくと $\displaystyle =2\int \dfrac{dt}{1-t^2}=\log \dfrac{1+w^2}{1-w^2}$ 次に， $2\mathcal{Re}(F(w))=F(w)+\overline{F(w)}=F(w)+F(\bar{w}),$ $2\mathcal{Im}(F(w))=F(w)-\overline{F(w)}=F(w)-F(\bar{w})$ を利用する．なお，ここで用いた $\overline{F(w)}=F(\bar{w})$ や複素数変数でも $e^ze^w=e^{(z+w)}$ や三角関数の加法定理が成り立つことを明らかではない． これは複素関数の解析接続の知識が必要なので後で解説する． $x(w)=\mathcal{Re}(F(w))=\arctan w+\arctan \bar{w}$ $\therefore \tan x=\dfrac{w+\bar{w}}{1-w\bar{w}}$ $y(w)=\mathcal{Re}(G(w))=\arctan (iw)+\arctan (-i\bar{w})$ $\therefore \tan y=\dfrac{i(w-\bar{w})}{1-w\bar{w}}$ $z(w)=\mathcal{Re}(H(w))=\dfrac{1}{2}\left(\log \dfrac{1+z^2}{1-z^2} +\log \dfrac{1+\bar{z}^2}{1-\bar{z}^2}\right)$ $=\dfrac{1}{2}\log \dfrac{(1+z^2)(1-\bar{z}^2)}{(1-z^2)(1-\bar{z}^2)}$ $1+\tan ^2 x=\dfrac{(z^2+1)(\bar{z}^2+1)}{(1-|z|^2)}$ $1+\tan ^2 y=\dfrac{(z^2-1)(\bar{z}^2-1)}{(1-|z|^2)}$ であるから $z=\log \dfrac{\cos y}{\cos x}$ $\therefore e^z=\dfrac{\cos y}{\cos x}$ シェルク曲面のグラフは次図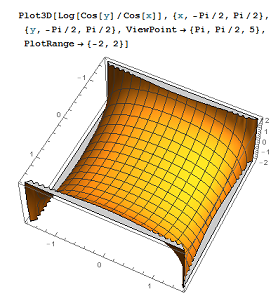． グラフは $z=\log \dfrac{\cos y}{\cos x}$ より $\cos x\cos y > 0$ の範囲の$x,y$であり共に$\frac{\pi}{2}+n\pi$を境に交互に同じ曲面が現れる．(右図) その曲面の一つ，$\dfrac{\pi}{2}\leqq x,y\leqq \frac{\pi}{2}$の範囲の曲面が左図． さて，この曲面で$x,y\to \dfrac{\pi}{2}-0$のときの$z$の極限を調べよう． $\bullet x=\dfrac{\pi}{2}-t^2,y=\dfrac{\pi}{2}-t$のとき $\dfrac{\cos y}{\cos x}=\dfrac{\sin t}{\sin t^2}\longrightarrow \infty \ \ (t\to +0)$ $\bullet x=\dfrac{\pi}{2}-t,y=\dfrac{\pi}{2}-t^2$のとき $\dfrac{\cos y}{\cos x}=\dfrac{\sin t^2}{\sin t}\longrightarrow 0 \ \ (t\to +0)$ $\bullet x=\dfrac{\pi}{2}-t,y=\dfrac{\pi}{2}-at$のとき $\dfrac{\cos y}{\cos x}=\dfrac{\sin at}{\sin t}\longrightarrow a \ \ (t\to +0)$ 以上のことは，$(x,y)\to (\frac{\pi}{1},\frac{\pi}{2})$のとき近づき方により$-\infty$から$\infty$ のどの値もとる．これは驚くべきことではなく，複素関数論からみれば真性特異点の持つ性質である． 次に虚数部分を求めよう． $\bar{x}(w)=\mathcal{Im}(F(w))$ より $i\bar{x}=\arctan w-\arctan \bar{w}$ $\therefore \tan (i\bar{x})=\dfrac{w-\bar{w}}{1+w\bar{w}}$ $\bar{y}(w)=\mathcal{Im}(G(w))=\dfrac{1}{i}(\arctan (iw)-\arctan (-i\bar{w}))$ $\therefore \tan i\bar{y}=\dfrac{i(w+\bar{w})}{1+w\bar{w}}$ $\bar{z}(w)=\mathcal{Im}(H(w))=\dfrac{1}{2}(\log \dfrac{1+w^2}{1-w^2} -\log \dfrac{1+\bar{w}^2}{1-\bar{w}^2})$ $=\dfrac{1}{2i}\dfrac{1}{2}\log \dfrac{(1+w^2)(1-\bar{w}^2)}{(1-w^2)(1+\bar{w}^2}$ $\bar{x},\bar{y},\bar{z}$の関係式を求めよう． $\cos (iw)=\cosh w,\sin (iw)=i\sinh w$ である． $1+(\dfrac{1}{\tan (iw)})^2=-\dfrac{1}{\sinh ^2w}$ であるから $-\dfrac{1}{\sinh ^2 \bar{x}}=1+(\dfrac{1}{\tan (i\bar{x})})^2$ $=\dfrac{(w^2+1)(\bar{w}^2+1)}{(w-\bar{w})^2}$ $-\dfrac{1}{\sinh ^2 \bar{y}}=\dfrac{-(w^2-1)(\bar{w}^2-1)}{(w+\bar{w})^2}$ $\therefore \sinh ^2\bar{x}\sinh ^2\bar{y} =-\dfrac{(w^2-\bar{w}^2)}{|w^2+1|^2|\bar{w}^2-1|^2} \ \cdots (1)$ 一方， $\bar{z}(w)=\mathcal{Im}(H(w))=\mathcal{Im}(\log \dfrac{1+w^2}{1-w^2})$ $=\arg (\dfrac{1+w^2}{1-w^2})$ したがって， $\sin \bar{z}=\mathcal{Im}\left(\dfrac{\dfrac{1+w^2}{1-w^2}}{\dfrac{|1+w^2|}{|1-w^2|}}\right)$ $=\dfrac{1}{i}\dfrac{w^2-\bar{w}^2}{|1+w^2||1-w^2|} \ \cdots (2)$ $(1),(2)$より $\sin \bar{z}=\sinh \bar{x}\sinh \bar{y}$ を得る． グラフは次図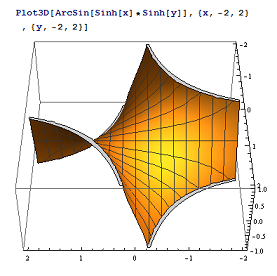． 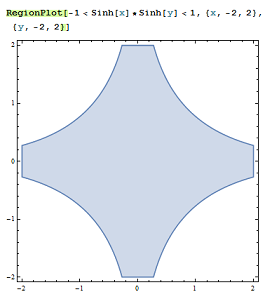共役曲面の定義域は $-1\leqq \sinh x\sinh y\leqq 1$ でその図が右図，左図が共役曲面のグラフである． シェルク極小曲面およびその共役曲面の第１基本量，第２基本量を計算しよう． なお，ここで求めたのは曲面上の$x,y,z$の関係であるあるから，等温座標系ではないし，もちろんこの表し方では， ２つの曲面の随伴曲面を作ることはできない． 曲面$z=f(x,y)$の第１基本量$E,F,G$，第２基本量$L,M,N$法線ベクトル$\boldsymbol{n}$は $E=1+f_x^2,F=f_xf_y,G=1+f_y^2$ $\boldsymbol{n}=\dfrac{(-f_x,-f_y,1)}{\sqrt{1+f_x^2+f_y^2}}$ $L=\dfrac{f_{xx}}{\sqrt{1+f_x^2+f_y^2}},M=\dfrac{f_{xy}}{\sqrt{1+f_x^2+f_y^2}}, L=\dfrac{f_{yy}}{\sqrt{1+f_x^2+f_y^2}}$ である．なお下付きの文字はその文字による偏微分である． シェルク極小曲面は $E=1+\tan ^2x,F=-\tan x\tan y,G=1+\tan ^2y$ $\boldsymbol{n}=\dfrac{(-\tan x,\tan y,1)}{\sqrt{1+\tan ^2x+\tan ^2y}}$ $L=\dfrac{1+\tan ^2 x}{\sqrt{1+\tan ^2x+\tan ^2y}}, M=0, L=\dfrac{1+\tan ^2 x}{\sqrt{1+\tan ^2x+\tan ^2y}}$ ガウス曲率$K$，平均曲率$H$は $K=\dfrac{-(1+\tan^2x)(1+\tan ^2y)}{(1+\tan ^2x+\tan ^2y)^2},H=0$ 共役曲面は $E=\dfrac{\cosh ^2y}{1-\sinh ^2x\sinh ^2y}$ $F=\dfrac{\cosh x\sinh x\cosh y\sinh y}{1-\sinh ^2x\sinh ^2y}$ $G=\dfrac{\cosh ^2x}{1-\sinh ^2x\sinh ^2y}$ 法線ベクトルを正規化すると複雑な式になる．極小曲面であることにとどめる． 曲面$z=f(x,y)$では $L:M:N=f_{xx}:f_{xy}:f_{yy}$ であるから $L:M:N=\cosh ^2y\sinh x\sinh y:\cosh x\cosh y:\cosh ^2x\sinh x\sinh y$ したがって，$EN-2FM+GL$を計算することより，$H=0$すなわち極小曲面であるある ことがわかる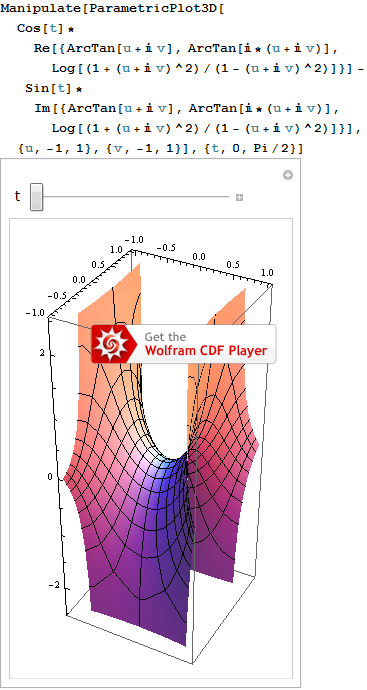． 右図は，シェルク曲面の随伴極小曲面である． シェルク曲面 $z=\log \dfrac{\cos y}{\cos x}$ は $z=f(x)+g(y)$ の形で表せる． 逆に $z=f(x)+g(y)$ の形の極小曲面を調べよう． すでに用いた曲面$z=h(x,y)$の第１基本量，第２基本量より $E=1+h_x^2=1+f'(x)^2,$ $F=h_xh_y=f'(x)g'(y),G=1+g'(y)^2$ $L=\dfrac{h_{xx}}{\sqrt{1+h_x^2+h_y^2}}$ $=\dfrac{f"(x)}{1+f'(x)^2+g'(x)^2},$ $M=\dfrac{h_{xy}}{\sqrt{1+h_x^2+h_y^2}}=0,$ $L=\dfrac{h_{yy}}{\sqrt{1+h_x^2+h_y^2}}=\dfrac{g"(y)}{1+f'(x)^2+g'(x)^2}$ $H=\dfrac{EN-2FM+GL}{2(EG-F^2)}=0$より $(1+f'(x)^2)g"(y)+(1+g'(y)^2)f"(x)=0$ $\dfrac{f"(x)}{1+f'(x)^2}=-\dfrac{g"(y)}{1+g'(y)^2}=a$ $\dfrac{f"(x)}{1+f'(x)^2}=a$ より， $f'(x)=\tan ax$ $\therefore f(x)=\dfrac{\log \cos(ax)}{a}$ 同様に $g(y)=-\dfrac{\log \cos(ay)}{a}$ したがって， $z=\dfrac{1}{a}\log \dfrac{\cos (ax)}{\cos (ay)}$ 続く 微分幾何